A strategy for structuring open inquiry and authentically assessing lab skills at the same.

How do we structure open inquiry?
My mantra is to always give students as little direction is possible without impeding productivity. I want them to figure things out. I want them to design experiments. I want them to make sense of their data at their own pace and in their own way. I want to scaffold as little as possible without them getting stuck. I have a few good strategies for tackling this and one of my favorites is by giving them a shell equation as a starting point, but even then, sometimes it can still be a bit too much for them to really feel like they are owning their work.
How do we authentically assess lab work without hampering collaboration?
We all want students to collaborate; to sit around whiteboard, thinking, discussing, planning, arguing etc... Still, at the end of the day, we have to assess these reasoning skills. A lab writeup can be okay, but how can we really tell where the collaboration ends and the independent thinking/writing/reflection began? Well, we can't.
I've been lucky enough to stumble into a lesson/assessment structure that I think tackles both of these questions with, what I think is tremendous success.
Here's the gist of the approach:
Students are given a brand new system about which they have little to no background knowledge.
Students design and conduct experiments to find relationships between variables in the system.
Students analyze their graphs, discuss end-behavior, and consider the necessary attributes mathematical models should have.
Students take a lab quiz using their data and logical reasoning to evaluate a proposed mathematical model for the system.
Tasks Sheets and Quizzes
If you want to dig right in to the materials, they are linked below. There is one for introducing conservation of momentum and another for introducing torque and angular acceleration.
Collision Inquiry
Rotation Inquiry
The First Attempt: Introduction to Collisions
It was the first day of our momentum unit. Students came in cold, without any knowledge of conservation of momentum (except perhaps from middle school). They were given the setup shown in the diagram below with the carts oriented so that they would stick together and collide inelastically upon impact. (Cart B begins at rest).
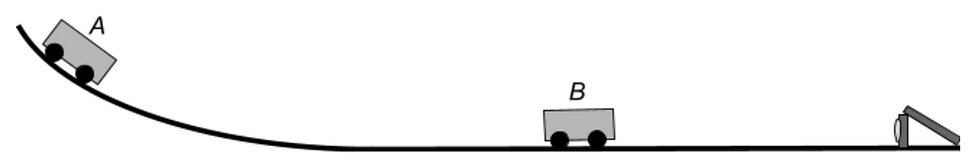
What was different about this lab though, was that students had no clear objective. They were given no procedural information or any tips on what variables to consider. I didn't give them any information about the system at all, not even a shell equation to get them thinking.
Instead, I told them the following:
This lab has no set goal or objective. You are tasked with collecting an abundance of data in order to make as much sense of the system as possible. Conduct experiments, make graphs, have discussions and seek out relationships between important variables. You likely will not be able to reach a clear definitive conclusion.
Okay... So they didn't have to turn in a report? There was no assessment of skills? They didn't have to submit a conclusion or even argue a claim?
Well actually, yes. They did have to do many those things, just in a different way. Rather than writing up work afterwards and turning it in, students were to spend time going over their results with their groups and making as much sense of them as possible. They then took an independent lab quiz on which they were allowed to use all of their data, graphs, and notes they took in class during the lab.
The quiz format was a bit different than what I've seen regarding lab quizzes in the past. On this quiz, I proposed a potential mathematical model for the system and students were tasked with using their results and logical reasoning to evaluate the proposed mathematical model. More on this later...
Side note: Physics teachers know that conservation of momentum for an inelastic equation is the mathematical model that describes this system and gives us all of the relationships between variables in this system and that is what the students are working toward. With that in mind, to keep us from getting bogged down with energy, I did tell them that we weren't particularly interested in the initial height of A as a variable and instead we should be focusing on just the collision. We then got into a discussion about how the speed of A just before colliding with B could be determined by removing B from the track and then measuring it with the motion detector. Then for the collision, B could then be placed back on the track and A released from that same height.
Looking at The Data: Some Juicy Discussions about End-Behavior
For two and a half class blocks (I know, it's a long time!), students discussed what variables to measure, designed their experiments, and gathered data. By this point in the year, students didn't struggle much in determining variables for their experiments. Within 20 minutes or so, all of my students had essentially determined that the following experiments should be conducted:
Collision Experiments
Independent Variable | Dependent Variable |
Cart A Initial Speed | Cart B Final Speed |
Cart A Mass | Cart B Final Speed |
Cart B Mass | Cart B Final Speed |
After gathering data, they made graphs in Logger Pro and began to argue about what kind of functions to fit to their data and this is where things got really juicy.
Now, if you've read some of my other posts, you might know that I LOVE to get students discussing the end-behavior of their graphs and this approach didn't disappoint!
Students quickly fell into discussions about whether trends should be linear, if they should increase forever, if they should have horizontal/vertical asymptotes, if there should be intercepts, etc... With a bit of prompting from me, this led them into discussions about how the system would behave at extreme ends of the range in their data collection and the end behavior. The following end-behaviors that came up are shown below:
End-Behaviors and Their Implications
mA >> mB | The final speed of the carts is the same as the initial speed of the carts. |
mB >> mA | The final speed of the carts should be zero. |
mB = 0 | The final speed should be equal to the initial speed of cart A. |
mA = 0 | The final speed should be zero. |
vi = 0 | The final speed should be zero. |
To be fair, not every student was able to make these connections. Many required support from their peers. Also, by this point in the year, I'd already gotten them to think about end-behavior on several occasions so this was definitely not a new skill for them.
These discussions led them to thinking about what functions might be good candidates to fit onto their graphs. For example, the implications of mA >> mB helped students understand that their vf vs. mA graph should have a horizontal intercept equal to vi.
It is important to note, however, that this end-behavior analysis is not enough for them to be 100% sure about what the right function is for each of their graphs, but this is actually a good thing! Not just good--it is essential! For the lab quiz, there needed to be enough ambiguity in their results that they were able to authentically evaluate a proposed mathematical model without knowing what the correct mathematical model should be.
The Lab Quiz
Before taking the lab quiz, students were given the following "pondering" to help them prepare and make sense of their data:
Ponderings: Things to think about and discuss with your group to prepare for the quiz.
What is the expected end-behavior of your graphs?
Consider how the system should behave when mA >> mB and when mB >> mA . How do these considerations relate to your graphs?
For one of your experiments, if one of the constant variables had been greater (or less) how would this have affected the shape of your graph?
Are there any types of equations that you can rule out immediately as being poor mathematical models? Are there any types of equations that could do a good job of modeling the system?
They were then required to bring in their data tables, graphs and any notes they took during the lab in discussions with their peers. The lab quiz consisted of two questions a short one and a longer one shown below. I'm not going to go through the short one.
Lab Quiz Question

So, let's unpack this a little bit. Using conservation of momentum we can see that the actual equation should look like this:

The equation I proposed in the question wasn't too far off from the actual one. We can also see that and vf does still indeed vary directly/indirectly with each corresponding variable in the correct way. The proposed model works pretty well, but breaks down when compared against student's vf vs. mA graph. In the model proposed in the question, vf increases infinitely as mA increases, but in the real equation, as mA goes to infinity, vf-->vi. This is an attribute that the students should have come across when discussing their end-behavior.
Students were not supposed to simply tell me whether the proposed model was right or wrong. Rather they were supposed to evaluate the equation using their data as well as the end-behavior implications. This was a wonderful way for me to see how well each student understood their data and to assess their ability to use data and logical reasoning to make meaningful claims. This rubric was used to assess their argument:
Lab Quiz Rubric
*Unfortunately, I handed all student work back before I scanned any, so I don't have any exemplary student work to show.
Final Thoughts
The first attempt at this was clearly a success, but there were some students who struggled. There was also a small group of students who clearly looked up conservation of momentum and then tried to explain what the right equation was without really using their data and end-behavior reasoning to evaluate the equation I proposed. This was despite the fact that I reminded them multiple times that we were doing a data-driven argument and that simply looking up the right equation wouldn't be of much use in this context. It took a while to really drive home the fact that we weren't really looking for THE RIGHT EQUATION and were instead looking to examine the validity of a proposed one.
Regarding their scores, I actually decided not to count the grade from the first quiz at all. After we did our second lab quiz (on rotations), I replaced the grade from the first one with the new one. I justified this based on the fact that these lab quizzes did not assess content at all. They were designed only to assess analysis and argumentation skills.
The second attempt went much smoother and it was clear that students had gotten the hang of this process. For the second attempt, we did an inquiry lab and quiz focused on looking at torque and angular acceleration. Here are links to the task sheet and the quiz.
My advice: Try it! Try it! Try it! Make it low stakes the first time, but then ramp it up a bit. Once students get the hang of it, they can really be in the driver's seat. I've always prided myself on being a teacher who focuses on student-centered learning, but this approach was on another level.

.png)
Comentarios